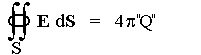Matter formed from emptiness; topological interpretation of electric charge

Appendix B
UNITARY FIELD THEORY AND QUANTUM GRAVITY
B.1. The process of unification in physics
B.2. Einstein's visions of geometric unitary field theory
B.3. Wheeler's geometrodynamics. Gravity and topology.
B.4. Quantum geometrodynamics
B.5. Gravitational field quantization
B.6. Unification of fundamental interactions. Supergravity. Superstrings.
B.7. General principles and perspectives of unitary field theory

B.3. Classical geometrodynamics. Gravity and topology.

In a sense, the successor of Einstein's efforts to create a unitary field theory, but in a slightly different way, became the American physicist John Archibald Wheeler, who, along with other collaborators, especially Ch.Misner, came from Einstein's original (verified and proven) general theory of relativity. He showed that to create a classic unitary field theory (while better than previous attempts) is not necessary in the general theory of relativity introduce any artificial and unjustified changes, it is enough to make full use of all geometric and topological possibility that general relativity provides.

In Chapter 2, we showed that the general theory of relativity overthrew space and time from the status of a kind of non-participating "scene" in which physical events take place, and made space-time a direct participant in physical events. According to GTR, the gravitational field is a manifestation of the curvature of empty spacetime - so we have a kind of "gravity without gravity"...

Electromagnetism "without electromagnetism"
As shown in §2.5, Einstein's gravitational field equation R_ik - ¹/₂ g_ikR = 8pT_ik have the important property that they not only describe the behavior of the gravitational field, but indirectly - via the laws of conservation of energy and momentum T^ik_;k = 0 - even its sources. Therefore, if we take the electromagnetic field in a vacuum, then from the Einstein equations the gravitational field excited by it

Maxwell's equations of this electromagnetic field F^ik _{; k} = 0 follow. If the curvature of spacetime is caused by an electromagnetic field, then the trace of the Einstein tensor on the left side (B.7) must be equal to zero, which gives R = 0 and the square of the Ricci tensor R^m_i R^k_m = d^k_i.(¹/₂ R_lmR^lm) is a multiple of the unit matrix.
  Einstein's and Maxwell's equations (which are systems of 2nd order equations) can therefore be combined into one system of 4th order equations - Einstein-Maxwell's equations, which in geometric form contains both Maxwell's electrodynamics (without charges) in curved spacetime and Einstein's equations indicating the curvature of spacetime by this electromagnetic field *). The electromagnetic field leaves characteristic "traces" on the geometry of space-time, from which it can be "recognized" and whose behavior is determined. The electromagnetic field (which is thus determined by the expression containing the roots of the Ricci tensor of the curvature R_ik) can thus be fully described using only gravitational quantities, ultimately using the components of the metric tensor g_ik. Maxwell's equations are then given by the relationship between Ricci's curvature and the rate at which this curvature changes; the laws of electrodynamics thus become pure geometric character. We get a kind of "electromagnetism without electromagnetism", in which the electromagnetic field is a manifestation of empty curved spacetime.
*) However, it turned out [281] that when integrating these Einstein-Maxwell equations, the respective boundary conditions (values of g_ik and their time partial derivatives g_{ik, 0}) on the initial Cauchy hypersurface x⁰ = 0 can correspond to more than one Maxwell field at the same time, more values of F_ik. The mentioned method of geometric description of electrodynamics thus becomes ambiguous in the general case ...

Misner and Wheeler (using Rainich's earlier results for the electromagnetic field in GTR [213]) therefore concluded that Einstein's original general theory of relativity already fulfilled what Einstein had tried unsuccessfully for the rest of his life: a unified description of gravitational and electromagnetic field. A description yet completely natural and spontaneous, in which no existing theories do not change in any way.

At the beginning of §B.1, we emphasized the unsatisfaction of the concept that the field is excited by a source different from the field. For the electromagnetic field as a source of gravity in a vacuum, the situation here has been successfully solved, at least in principle. The "material" sources of the electromagnetic field are electric charges. Here, too, geometrodynamics proposes an elegant solution: the topological interpretation of electric charges by means of some "tunnels" in a curved space, which capture electric lines of force and effectively behave like electric charges (see below "Topological interpretation of electric charge").
  The source of gravity in classical physics is mainly general, unspecified and unstructured matter - objects (bodies, particles) having mass. In previous unitary theories the particles as trying to interpret some particularities (singularities) in the field, which leads to many difficulties (see §3.7), or as a some continuous densified structures having their laws of internal motion; however, these laws of internal motion were introduced from the outside, and it was not clear how to derive them within a closed theory.
  It is different in geometrodynamics. If we take Schwarzschild's geometry in empty asymptotically planar spacetime, the ordinary law of gravity will apply at great distances from the center as if there were real matter in the center. It is thus an empty curved space in which gravitational attraction acts, so Schwarzschild's geometry is the simplest geometrodynamic model of "matter without matter". However, it is a artifical model with a topology different from the Euclidean topology and contains a singularity (§3.4).

Matter "without matter"
Geons
However, the laws of general theory of relativity allow the existence of objects with the usual Euclidean topology and without singularities, behaving like real matter (exciting the gravitational field and responding to this field), and these objects are composed purely of the field itself. When electromagnetic waves propagate through space, they excite a gravitational field around them - they curve the space-time in which they propagate, and this does not remain without affecting their motion. According to the general theory of relativity, very powerful electromagnetic waves can create such a strong gravitational field around them, that they will be forced to permanently move along the closed paths. The electromagnetic waves thus create a kind of gravitational "waveguide" curved around this geometry of spacetime (from the gravitational field) in which they circulate permanently - Fig.B.2a. Such a formation of electromagnetic waves, held together by the gravity of its own field energy, is called a (electromagnetic) geon [284].

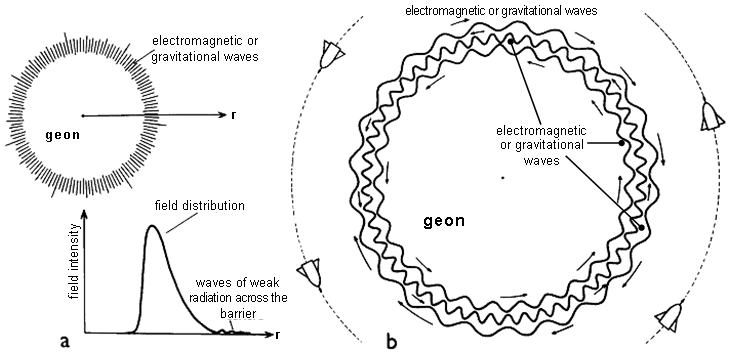
Fig.B.2. Massive electromagnetic or gravitational waves can create such a strong gravitational field (curvature space-time) around them, that they will be permanently forced to circulate in a closed "gravitational waveguide" - a metastable material formation geon is created. a) Average field distribution in the geon.
b) Due to its gravitational effects, the geon behaves like any other matter (even a planet) - we can, for example, put a satellite into orbit around a geon.

The geon can be reached, for example, by the following imaginary experiment: Let us have a black hole of mass M, to whose photon sphere r = 3M (§4.3) we will send massive doses of electromagnetic waves. With the increasing amount of energy of electromagnetic waves moving on photon sphere, these waves will increasingly contribute to the overall gravitational field that keeps them there, so we can reduce the weight of the black hole by the appropriate value (in reality, however, it is prohibited by the 2.law of dynamics of black holes - see §4.6). With a sufficiently large accumulation of electromagnetic waves, we can completely "remove" the black hole *), because the energy ~ mass of the waves themselves is enough to create a sufficiently strong gravitational field to keep itself on the "photon sphere" - to maintain the circulation of waves in the geon.
*) However, no mechanism is known for removing (or gradually "controlled removal") the initiating black hole inside the genon (apart from the hypothetical or sci-fi topological tunnel "wormholes" - cf. §4.4, passage "Wormholes - bridges to other universes? Time machines?"). This is just an artificial imaginary experiment. In a "natural" way, perhaps geons could arise in massive primordial fluctuations in Planck's time at the beginning of the universe..?.. - cf. §5.5 "Microphysics and cosmology. Inflationary universe.".
  If the geon of total mass M is spherically symmetric, it will evoke a spherically symmetric gravitational field and the space-time metric will be (compare with §3.4)  

ds ²   =   - g _tt dt ² + g _rr dr ² + r ² (d J ² + sin ² J d j ² ).

The radial component of the metric has the usual Schwarzschild form g_rr = 1/[1 - 2m(r)/r], where m(r) is the mass~energy contained inside a sphere of radius r. The time component of the metric outside the geon also has the Schwarzschild form g_tt = 1 - 2M/r, inside the geon it has the value g_tt = 1/9 (the time inside the geon flows three times slower than far from the geon). The geon is not completely stable, but only metastable - part of the energy of the waves penetrates through the centrifugal and gravitational barriers, the geon slowly dissolves (the slower the greater the number of wavelengths around the circumference), or vice versa, it could collapse to form a black hole. For a distant observer, the geon will have the same gravitational effects as any other matter (such as a planet) - we can, for example, orbit a satellite into orbit around a geon (Fig.B.2b).
  Such a mass composed of electromagnetic waves may seem strange to us, but the material nature of electromagnetic waves is sufficiently established. We get an even more suggestive picture when we replace electromagnetic waves with gravitational waves. Gravitational waves also transfer energy (§2.7 and 2.8), curved space-time (universal excitation of gravity) and according to the general theory of relativity, they can also create a "gravitational" geon, which will externally manifest itself as real matter by its gravitational effects .
  But what are gravitational waves? - are waves of the gravitational field, ie fluctuations in the geometry of empty spacetime. The external observer thus witnesses an interesting thing: the rippling curvature of empty space-time "without matter" will appear as a material formation! The gravitational geon is thus an illustrative model of a kind of "matter without matter", matter formed literally from the "emptiness" of space with rippling curvature.
  Here it must be said that the whole concept of geometrodynamics meets certain philosophical- methodological logical problems; however, this does not mean that the above concepts contradict the basic postulates of materialist philosophy *), which is a natural platform for physics and science in general.
*) If we observe matter either on ever smaller scales of the microworld or, conversely, on ever larger scales of the megasworld, matter will gradually lose some attributes to which we are accustomed from the common experience of our macroworld, and eventually new attributes will begin to appear. However, the basic feature of matter always remains - to be an objective reality.

However, the hypothetical (model) geon is only a certain extreme example of the construction of a material object from the geometry of spacetime; above were mentioned some fundamental problems with its formation and with the stability of the geone against the radiation and scattering of gravitationally trapped orbiting waves-quantums of electromagnetic or gravitational waves. The initial hopes that the geon might be used as a classical model of stable (elementary?) Particles were not fulfilled. It is a product of classical field theory, and until a fully satisfactory quantum theory of gravity is built, the possible relationship between geons and quantum-mechanical elementary particles cannot be specified. There is no hope yet that there might be some "micro-geons" stabilized by quantum effects (omehow, like the orbits of electrons in atomic shells, are stabilized by quantum corpuscular-wave dualism?), which could model elementary particles ...
  From a theoretical point of view, we don't even have to go to such complex constructions. In fact, every gravitational wave described by its Isaacson tensor of nonlocal energy-momentum (see §2.8) is such a "matter without matter", composed of a "vacuum" (understood in the usual sense). The fenomenon, as even in "empty" space without the usual "real" material sources, appear kind of effective mass having global gravitational effects, is, after all, a similar situation in electrodynamics, where even in a vacuum without the presence of electric charges (and currents) for a non-stationary electromagnetic field, a Maxwell shear current appears, having magnetic effects the same as the "real" current of electric charges ...

Topological interpretation of electric charge
Charge "without charge"
Now let's notice the electric charges. Electric charges (and their currents) are the sources of the electromagnetic field, but they are also something "foreign" in the theory of the electromagnetic field itself - a kind of substance different from the field. In places of positive electric charges, electric lines of force begin and exit on all sides, in places of negative electric charges, lines of force enter and end there from all sides (Fig.B.3a); Maxwell's field equations do not apply here. According to Gauss's theorem, the total electric charge in any part of space can be determined by surrounding the investigated area with an (imaginary) closed surface S and measuring the intensity E electric field in all places of this closed area - we determine the "number of lines of force" that go in or out (Fig.B.3a).

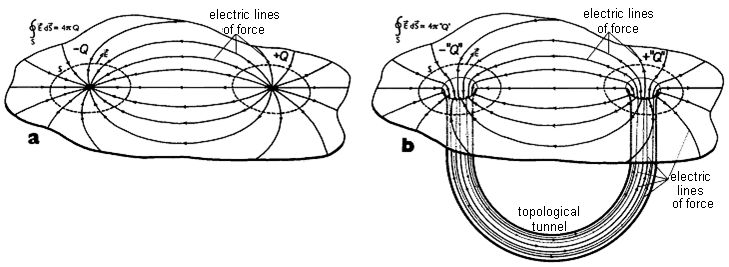
Fig.B.3. Classical and topological interpretation of electric charges.
a) The usual understanding of electric charge Q as "substance", from which the field lines of the excited electric field originate (or enter).
b) Topological interpretation of electric charge - there is no "real" charge as a substance, the lines of force do not begin or end anywhere, they are just captured and pass through a topological tunnel, whose throats then appear as "apparent" charges "Q".

But can't the lines of force that go in, somehow "unnoticed" get out again without noticing it on the enclosed area bounding this interior (or similarly, the lines of force that go out to get back in)? At first glance, such a question seems absurd. If we lock someone in jail on all sides, acording to common sense is unthinkable to get out, without having to go through the wall of his prison - to break through the wall, open the door in it.
  Let's draw this situation in a two-dimensional case on a piece of paper; instead of humans we think of ants, which we will consider here as two-dimensional beings (Fig.B.4). In Fig.B.4a, the two-dimensional world of ants has the usual characteristics, and a prisoner inside a closed curve cannot really get out without passing through this boundary of his prison.
  

Fig.B.4. Influence of topological properties of space on the possibilities of movement.
a) A prisoner (an ant) surrounded on all sides by a prison wall cannot get out in a space (here two-dimensional) with the usual topological characteristics without passing through the prison wall.
b) In an area with multiple continuous topologies, it is possible to leave a closed prison without having to go through its wall. The ant can walk through the topological tunnel and look at the intact wall of his prison from the outside.

But what if the two-dimensional world of ants looks topologically as shown in Figure B.4b? An ant trapped in an area surrounded on all sides by a closed curve (prison wall) can go through a "tunnel" and look at its prison from the outside without having to go through a closed wall of its prison at some point. From the point of view of the three-dimensional environment, in which this construction is embedded, there is nothing strange about it - the ant, although it still moves within its two-dimensional surface (its world), "creeps or overcomes" the wall of its prison, so to speak, through "another dimension". From the point of view of the two-dimensional ants themselves, for which no "third dimension" exists, however, a miracle happened: a prisoner surrounded on all sides by a wall suddenly came from somewhere outside to look at the intact wall of his prison! The reason is that said two-dimensional space has different topological properties than in Fig.B.4a. It is multiple continuous. The closed curve here no longer has to be the boundary of the area inside! The local geometric properties at each location while doing so can be quite common (only slight curvature).

Now we can return to electric charges. In Fig.B.3a, a positive and a negative electric charge are shown in the usual way in a two-dimensional drawing - the lines of force emerge from the positive charge (according to the agreement) and end at a negative charge. If we surround the charge with an imaginary closed surface S, we can "count" the force lines entering or leaving to determine the value of the charge Q inside. However, there may not be any "real" electric charge here! With a suitable topology of the space, as shown in Fig.B.3b, the force lines will enter inside the closed area S, but they will not end there, but they will pass through a topological "tunnel" to another place in the space, from where they will come out again and return. To the external observer, who will measure the electric field, one "tunnel mouth" will appear as a negative charge -"Q" (with the lines of the line going in) and the other throat of the tunnel as a positive charge +"Q" (the lines of force go out). The electric field, whose lines of force pass through a topological tunnel, satisfies Maxwell's equations everywhere. Consequently, the total flux of the electric field intensity trough the mouth of the tunnel cannot change over time, if the topology is not changed; it does not matter the variability of the electromagnetic field, the curvature of space, changes in the "cross-section" of the topological tunnel or the distance between its two mouths. Electric field flow through each closed surface S surrounding the tunnel

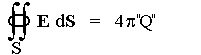
thus it complies with the law of conservation of electric charge and the Gauss theorem of electrostatics.

Such a topological interpretation of an electric charge is actually a "charge without charge": no "real" electric charges exist here, electric lines have no beginnings or ends, they are only captured and pass through a topological tunnel of space, the individual mouths of which then appear as positive and negative charges "Q". Thus, a free electromagnetic field in a vacuum without charges can generate (effective) electric charges due to the suitable topological structure of the space. In this theory, electric charge appears as a nonlocal property of electrodynamics (without sources) in multiple continuous spaces.

Is there only one electron? - "One electron universe" ?
Elementary carriers of negative electric charge are electrons (and positive positrons, protons in atomic nuclei and quarks inside them) - Elementary particles and their properties. All electrons are exactly the same, indistinguishable; they have the same electric charge and the same mass. We take it as a physical fact. But is it possible to think about why this is so? We don't know. Although geometrodynamics does not directly describe electrons, we can mention here one hypothesis that tried to explain it, and its author is the founder of geometrodynamics. In 1940, J.A. Wheeler came up with the hypothesis that there is only one electron in the universe, which has such a bizarrely "disturbed" and multiple trajectory in space-time that it intersects the hypersurface of the present in a huge number (perhaps 10⁸⁰) of points that represent all the individual electrons finding currently in space. That's why all these electrons are identical, because it's actually the same electron...
The main shortcoming of this hypothesis is the assumption of world lines of electron movement outside the light cone, "zig zag" into the future and into the past, which the theory of relativity does not allow (the interpretation of antiparticles using the movement of particles into the past is now abandoned). It was just an interesting bizarre idea that was not considered real even by its authors...

B.2. Einstein's visions of geometric unitary field theory		B.4. Quantum geometrodynamics

Vojtech Ullmann