Local principle of equivalence and its consequences

AstroNuclPhysics ® Nuclear Physics - Astrophysics - Cosmology - Philosophy

As we showed in the previous §2.2, Einstein fully understood and generalized the universality of gravitational action on all physical phenomena in the principle of equivalence, which is the basis of the general theory of relativity :

The gravitational field at each point is locally equivalent (for all physical processes) to a situation where there is no gravitational field, but the reference system (observer) at this point moves with the appropriate acceleration - it is non-inertial .

A homogeneous gravitational field can be fully "imitated" by a kinematic field of inertial forces in a non-inertial frame of reference; in this case, it is always possible to find a frame of reference in which the free bodies move as if there were no field. For real (inhomogeneous - permanent) gravitational fields this is no longer possible, because there is no reference system canceling the intensity of such a field at all points. However, in a sufficiently small vicinity of each point, the gravitational field of any origin and structure can be considered homogeneous, and thus kinematic.

In other words, gravitational and inertial forces have the same physical properties. The mysterious gravitational forces have thus been described by means of apparent inertial forces, which can already be dealt with by special theories of relativity (as shown in §2.1). The local principle of equivalence is thus a connecting bridge between gravitational and non-gravitational physics.

A direct consequence of the universality of gravitational action is the possibility to always and in any place introduce a locally inertial system - a frame of reference connected to a body free "falling" in a gravitational field (eg a cabin of a free-moving satellite) - in which there is a state of "weightlessness" for all physical processes. And in any strong gravitational field, even inside a black hole! The size of this local inertial system, however, depends on how homogenous is the given gravitational field, so that we can neglect with desired accuracy affect the inhomogeneity in the appropriate place. For a completely homogeneous field, this size is unlimited - the locally inertial system is also a global inertial system. A homogenous gravitational field, giving all bodies everywhere the same acceleration, is therefore not physically distinguishable from the apparent field arising without the presence of gravity in a uniformly accelerated frame of reference, so it is not observable at all *). Conversely, in a strongly inhomogeneous gravitational field (eg, deep inside a black hole near a singularity), the locally inertial system may already be so small that it does not even contain the dimensions of the elementary particle. Finally, in the singularity of spacetime itself (see §3.7) it is no longer possible to introduce a locally inertial system, because there are no regular reference systems, no physical measurements can be performed here, there is no regular spacetime. This is also the only case limiting the applicability of the equivalence principle.
*) It is similar to the potential in electrostatics, where an electric field having the same non-zero potential everywhere is zero, and changing the potential at all places by the same value does not change the existing electric field in any way; the actual field strength is given by the potential gradient. Similarly, the actual gravitational field differs from the purely kinematic field of "apparent forces" in its inhomogeneity.

Thus, we may make a seemingly somewhat paradoxical sounding statement: "The existence of a state of weightlessness is a fundamental characteristic properties of gravity - of weight." However, this is indeed the case, because we do not encounter anything similar in any other physical field. Since in the local inertial system there is a perfect state of weightlessness in the infinitesimal surroundings of its origin and gravitational forces do not exist for any physical process, all physical phenomena will occur locally according to the laws of nongravity physics, ie according to the laws of special theory of relativity without gravitational field. This is actually the principle of equivalence expressed in other words.

The process of finding physical laws in the presence of a gravitational field according to this concept therefore consists of the following four stages :

a) Divide spacetime into sufficiently small areas within which the gravitational field can be considered homogeneous.

b) For each such region, introduce a locally inertial frame of reference and apply the laws of special theory of relativity according to the principle of equivalence.

c) Appropriate transformation of space-time coordinates in these laws go to the corresponding non-inertial frame of reference, in which the resulting "fictitious" forces will be locally identical to the actual gravitational forces at a given location (for a given initial frame of reference).

In other words, the problem of determining the influence of gravity on physical processes is decomposed into a number of local problems that can be solved on the basis of the principle of equivalence within the special theory of relativity. How this program is implemented in practice for individual laws of physics will be outlined in the following paragraph. Here we will only show the connections between gravity and the properties of space-time to the principle of equivalence.

Because the path of a body in a gravitational field does not depend at all on the mass, shape or structure of the body, this trajectory is a characteristic of a given (surrounding) spacetime rather than the observed body. The principle of equivalence shows that the gravitational field can be exhaustively described (for all physical phenomena) locally using kinematics. The term that expresses the universal physical properties of bodies (kinematics) and the course of physical processes in general, is space-time, its geometric properties. The statement "every body in its surroundings, through gravity, universally affects all physical processes" is equivalent to the statement: "this body in its surroundings influences the properties of spacetime". In other words, everything will be de look exactly the same when, instead of the gravitational field and investigating its effect on the laws of physics, we consider the geometric structure of spacetime without the gravitational field and observe the connections between the laws of physics and the properties of spacetime. The logical chain gravity ® kinematics ® spacetime thus leads to the interpretation of gravity as a manifestation of the geometric properties of spacetime, in fact to the identification of both concepts *).
*) Some physicists (and philosophers) [185] disagree with such an identification and consider the curvature of spacetime to be just one manifestation of gravity. However, a consistent conclusion from the principle of equivalence shows that the gravitational field is indeed nothing more than a curved spacetime (see also "Appendix B" on unitary field theory).

In Minkowski spacetime STR the laws of (pseudo) Euclidean geometry apply, it is planar spacetime (flat). There are global inertial systems that move uniformly in a straight line; each locally inertial frame of reference is automatically and globally inertial. In inhomogeneous gravitational field (around the gravitational body) however, local inertiality is reached at each point by acceleration in general another magnitude and direction, so that such locally inertial systems can not combine in to global inertial frame of reference.

The connections between the globally inertial, non-inertial and locally inertial system in the presence of gravity can be clearly shown by a simple imaginary experiment according to Fig.2.5. From the "inertial" system S, we send two rockets A and B , which after motors shutdown will continue to move by inertia. Somewhere at a great distance, the rocket A spontaneously (perhaps due to a fault in the on-board electronics) starts the side control motor and leaves it switched on, so that the rocket will move unevenly - in a circular path. Let the rocket B get somewhere far from the place of its launch gets into the gravitational action of a very massive body M (perhaps a planet or an extinct star), around which it begins to orbit in a circular orbit. An observer from the "inertial" system S (for which he thinks that is globally inertial) notices, e.g. by analyzing radio signals, that both rockets began to move non-inertial - along closed paths. He will fly after them to look.

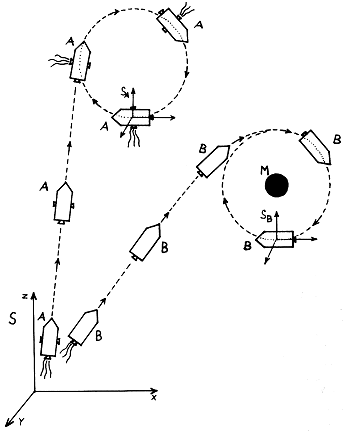Fig.2.5. On the relationship between inertial, non-inertial and locally inertial frame of reference.
Rockets A and B , which in the imaginary experiment start from the "inertial" frame of reference S , begin to move non-inertially with respect to it over time. For rockets A causes switch on the side of the engine, the rocket B is captured into orbit around graviting body M . The reference system S_A is non-inertial, but the system S_B is locally inertial; therefore in the presence of gravitational bodies neither the system S can be considered globally inertial.

First, it arrives at rocket A , enters it and easily understands the cause of its uneven movement: the side engine is switched on, which exerts a "pull" force perpendicular to the direction of movement. The reference system S_A associated with this rocket is non-inertial (free bodies will move relative to it with acceleration); the same would apply if the cause were not the engine running, but perhaps a very strongly electrically charged body around which the oppositely charged rocket would orbit - here bodies with a specific charge different from the specific charge of the rocket would move with respect to it with acceleration. Then the observer flies to rocket B and enters it. Here, however, they do not see any cause of the uneven motion and are surprised to find that the system S_B associated with this rocket is (locally) inertial: there is a "state of weightlessness", all free bodies in the rocket cabin move evenly, the accelerometer shows a zero value. This is due to the universality of the gravitational action of the body M, as shown in the previous paragraph.

The case of rocket A is fine, the cause of the uneven motion is a non-universal force, the frame of reference S_A associated with rocket A is non-inertial, while the system S can still be considered inertial. It is different with the B rocket. The reference frame S_B connected to it is locally inertial, but it moves unevenly with respect to the original "inertial" system S ! In a global inertial system, however, all locally inertial systems (which are also globally inertial) must move evenly with respect to each other. Thus we see that no reference system S in which there are gravitational bodies can no longer be globally inertial, because the individual locally inertial systems can move relative to each other with acceleration. Global inertial system here do not exists. The fact that in an inhomogeneous gravitational field it is not possible to combine individual locally inertial reference frames into a globally inertial system shows that spacetime is no longer planar (as in STR), but is curved.

In §2.1 it was shown that the field of fictitious inertial forces arising in non-inertial frames of reference can be described by the metric tensor g_ik , which then appears in generally invariant laws of physics. The difference between the field of "apparent" inertial forces (eg centrifugal and Coriolis forces in a rotating frame of reference) and the "right" gravitational field is only that in the first case spacetime is flat and a suitable transformation can return to the global inertial system, while in the latter case not. This is only a global difference, there is no difference locally. Because the laws of physics are local in nature, we can infer that the actual gravitational field together with a field of "fictitious" inertia forces in any frame of reference is fully determined and described by the metric tensor of spacetime g_ik .

A similar attitude to the seemingly fundamental difference between inertial forces (which arise from the accelerated motion of a frame of reference - and again disappear on transition to a suitable inertial system) and real gravitational forces which cannot be canceled throughout space by transformation of a frame of reference, has a close analogy in magnetism. The magnetic field around permanent magnets or conductors of electric current in its entirety can not cancel coordinate transformations; in this sense, such a magnetic field resembles a gravitational field around material bodies. In contrast, the magnetic field created by the movement of a charged body disappear during the transition to the reference system moving together with the body (there remains only the electrostatic field). Also, the magnetic field induced by the convective current of charges moving at the same speed can be annulled globally by switching to a reference system moving at the same speed as the charges flowing. However, the same laws apply to magnetic fields excited by convection currents as well as to magnetic fields around permanent magnets and conduction currents, and therefore there is no reason to consider them physically different. Likewise, there is no reason to physically distinguish the "apparent" field of inertial forces from the "real" gravitational field around material bodies.

To summarize, in any gravitational field it is possible to introduce a number of locally inertial reference frames (at each point) in which there is a state of weightlessness and all physical processes in them take place locally according to the laws of special theory of relativity without gravity. In the real (inhomogeneous) gravitational field, individual locally inertial systems will have different acceleration and thuse variable mutual velocities, so according to the Lorentz transformation, there will be different spatial relations and a different course of time in various places. However individual local inertial systems generally can not be joined into one global inertial system - spacetime is not planar Euclidean but curved Riemannian. The universality of gravitational action expressed in the principle of equivalence thus leads to deep connections between by gravity and geometry :

Gravity, black holes and space-time physics :
Gravity in physics	General theory of relativity	Geometry and topology
Black holes	Relativistic cosmology	Unitary field theory
Anthropic principle or cosmic God
Nuclear physics and physics of ionizing radiation
AstroNuclPhysics ® Nuclear Physics - Astrophysics - Cosmology - Philosophy



2.2. Versatility of gravity		2.4. Physical laws in curved spacetime

Principle of equivalence - the gravitational field is a manifestation of the curvature of spacetime